Online Talks

Since the revolutionary first discovery of a planet that orbits a Sun-like star outside the solar system, astronomers have detected and partially characterised several thousand extrasolar planets. These planets have a diverse range of masses and radii, consisting of some that are as small as Earth, and some that are much bigger than Jupiter. Some planets orbit their stars very closely, much more closely than Mercury orbits our Sun. In this talk, I will introduce some of the remarkable observational discoveries of extrasolar planets over the past two decades, before describing how Mathematics can be used to understand some of their properties.

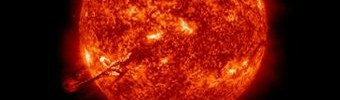
Our nearest star, the Sun, is an active star, with solar activity leading to powerful events like solar flares and ejections of hot plasma that can be shot in the direction of Earth. Solar activity waxes and wanes in an eleven year cycle and even switches off occasionally. In this talk I will explain the mathematics behind the solar cycle, what it has to do with chaos and the double pendulum and what effect solar variability may have on the climate.

It is possible to shuffle a pack of cards so that the cards from two halves are perfectly alternated. While this shuffle looks perfectly fair, it is almost as unfair as you can get. The shuffler can work out the destination of each card and, with a bit of thought, can deal winning hands of cards effortlessly. The possibilities in cheating and in card magic are immense! In this talk we will look at this “perfect” shuffle and the interesting mathematics behind it. (Involves audience participation.)

A million dollars is on offer for each of six mathematical problems. It used to be seven, but Grigori Perelman, solved one of them, the Poincare Conjecture. Surprisingly he has refused to take the million dollar prize!
In this talk I will look at the some of these problems, why they are important and their relevance to the real world. The problems cover subjects such as computers, fluids, and prime numbers. (Involves audience participation.)

From searching the internet to managing a manufacturing company, everyone knows that maths plays a central role in today’s hi-tech civilisation. But what sort of maths? In this talk we’ll meet a few familiar ideas from algebra and geometry which seem simple and elegant on first sight. But when massively scaled up and implemented on powerful computers, we’ll see how these techniques have truly changed the world.

Why does jelly wobble? Why do rubber balls bounce? And how do opals form? The answers to all of these questions lie in combinatorics. Simply by counting the different ways to arrange their molecules, you can understand the behaviour of many complex materials.

We discuss the ways in which Einstein’s special theory of relativity imposes a limit on the speed of motion, and just how much of a barrier it is.

Many of the materials we encounter on a daily basis – gloopy substances such as shower gel, pizza cheese, tomato ketchup, plastics or paint – exhibit flow properties that seem halfway between that of a liquid and a solid. This talk (illustrated by a number of demonstrations) gives an introduction to the rich variety of flow phenomena that are possible, and to the mathematics that can be used to describe them.

Infinity has intrigued mathematicians and philosophers for centuries. But in the 19th century, Georg Cantor began the first rigorous analysis of how infinite collections of objects behave. His discoveries would change the subject forever, and one problem that he couldn’t solve would go on to shake the foundations of mathematics in the 20th century.

Suitable for students in KS5